6.7: Tabulated Enthalpy Values

The enthalpy changes for many types of chemical and physical processes are available in the reference literature, including those for combustion reactions, phase transitions, and formation reactions. As we discuss these quantities, it is important to pay attention to the extensive nature of enthalpy and enthalpy changes. Since the enthalpy change for a given reaction is proportional to the amounts of substances involved, it may be reported on that basis (i.e., as the ΔH for specific amounts of reactants). However, we often find it more useful to divide one extensive property (ΔH) by another (amount of substance), and report a per-amount intensive value of ΔH, often “normalized” to a per-mole basis. (Note that this is similar to determining the intensive property specific heat from the extensive property heat capacity, as seen previously.) A standard state is a commonly accepted set of conditions used as a reference point for the determination of properties under other different conditions. For chemists, the IUPAC standard state refers to materials under a pressure of 1 bar and solutions at 1 M, and does not specify a temperature (it used too). Many thermochemical tables list values with a standard state of 1 atm. Because the ΔH of a reaction changes very little with such small changes in pressure (1 bar = 0.987 atm), ΔH values (except for the most precisely measured values) are essentially the same under both sets of standard conditions. We will include a superscripted “o” in the enthalpy change symbol to designate standard state. Since the usual (but not technically standard) temperature is 298.15 K, we will use a subscripted “298” to designate this temperature. Thus, the symbol (\(ΔH^\circ_\)) is used to indicate an enthalpy change for a process occurring under these conditions. (The symbol ΔH is used to indicate an enthalpy change for a reaction occurring under nonstandard conditions.)

Enthalpy of Combustion

Standard enthalpy of combustio n (\(ΔH_C^\circ\)) is the enthalpy change when 1 mole of a substance burns (combines vigorously with oxygen) under standard state conditions; it is sometimes called “heat of combustion.” For example, the enthalpy of combustion of ethanol, −1366.8 kJ/mol, is the amount of heat produced when one mole of ethanol undergoes complete combustion at 25 °C and 1 atmosphere pressure, yielding products also at 25 °C and 1 atm. \[\ce(l)+\ce(g)⟶\ce+\ce(l)\hspaceΔH_^\circ=\mathrm\] Enthalpies of combustion for many substances have been measured; a few of these are listed in Table \(\PageIndex\). Many readily available substances with large enthalpies of combustion are used as fuels, including hydrogen, carbon (as coal or charcoal), and hydrocarbons (compounds containing only hydrogen and carbon), such as methane, propane, and the major components of gasoline.

Example \(\PageIndex<3>\): Using Enthalpy of Combustion As Figure \(\PageIndex<3>\) suggests, the combustion of gasoline is a highly exothermic process. Let us determine the approximate amount of heat produced by burning 1.00 L of gasoline, assuming the enthalpy of combustion of gasoline is the same as that of isooctane, a common component of gasoline. The density of isooctane is 0.692 g/mL. Figure \(\PageIndex<3>\): The combustion of gasoline is very exothermic. (credit: modification of work by “AlexEagle”/Flickr) Solution Starting with a known amount (1.00 L of isooctane), we can perform conversions between units until we arrive at the desired amount of heat or energy. The enthalpy of combustion of isooctane provides one of the necessary conversions. Table \(\PageIndex\) gives this value as −5460 kJ per 1 mole of isooctane (C₈H₁₈). Using these data,

Exercise \(\PageIndex<3>\) How much heat is produced by the combustion of 125 g of acetylene? Answer 6.25 × 10 3 kJ

Emerging Algae-Based Energy Technologies (Biofuels) As reserves of fossil fuels diminish and become more costly to extract, the search is ongoing for replacement fuel sources for the future. Among the most promising biofuels are those derived from algae (Figure \(\PageIndex<4>\)). The species of algae used are nontoxic, biodegradable, and among the world’s fastest growing organisms. About 50% of algal weight is oil, which can be readily converted into fuel such as biodiesel. Algae can yield 26,000 gallons of biofuel per hectare—much more energy per acre than other crops. Some strains of algae can flourish in brackish water that is not usable for growing other crops. Algae can produce biodiesel, biogasoline, ethanol, butanol, methane, and even jet fuel. According to the US Department of Energy, only 39,000 square kilometers (about 0.4% of the land mass of the US or less than \(\dfrac\) of the area used to grow corn) can produce enough algal fuel to replace all the petroleum-based fuel used in the US. The cost of algal fuels is becoming more competitive—for instance, the US Air Force is producing jet fuel from algae at a total cost of under $5 per gallon. The process used to produce algal fuel is as follows: grow the algae (which use sunlight as their energy source and CO₂ as a raw material); harvest the algae; extract the fuel compounds (or precursor compounds); process as necessary (e.g., perform a transesterification reaction to make biodiesel); purify; and distribute (Figure \(\PageIndex\)).

Standard Enthalpy of Formation

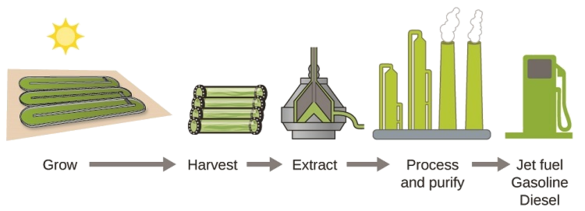
A standard enthalpy of formation \(ΔH^\circ_\ce\) is an enthalpy change for a reaction in which exactly 1 mole of a pure substance is formed from free elements in their most stable states under standard state conditions. These values are especially useful for computing or predicting enthalpy changes for chemical reactions that are impractical or dangerous to carry out, or for processes for which it is difficult to make measurements. If we have values for the appropriate standard enthalpies of formation, we can determine the enthalpy change for any reaction, which we will practice in the next section on Hess’s law. The standard enthalpy of formation of CO₂(g) is −393.5 kJ/mol. This is the enthalpy change for the exothermic reaction: \[\ce(s)+\ce(g)⟶\ce(g)\hspaceΔH^\circ_\ce=ΔH^\circ_=−393.5\:\ce \] starting with the reactants at a pressure of 1 atm and 25 °C (with the carbon present as graphite, the most stable form of carbon under these conditions) and ending with one mole of CO₂, also at 1 atm and 25 °C. For nitrogen dioxide, \(\ce_\), \(ΔH^\circ_\ce\) is 33.2 kJ/mol. This is the enthalpy change for the reaction: \[\frac\ce(g)+\ce(g)⟶\ce(g)\hspaceΔH^\circ_\ce=ΔH^\circ_=+33.2\: \ce \] A reaction equation with \(\frac\) mole of N₂ and 1 mole of O₂ is correct in this case because the standard enthalpy of formation always refers to 1 mole of product, NO₂(g). You will find a table of standard enthalpies of formation of many common substances in Tables T1 and T2. These values indicate that formation reactions range from highly exothermic (such as −2984 kJ/mol for the formation of P₄O₁₀) to strongly endothermic (such as +226.7 kJ/mol for the formation of acetylene, C₂H₂). By definition, the standard enthalpy of formation of an element in its most stable form is equal to zero under standard conditions, which is 1 atm for gases and 1 M for solutions.

Example \(\PageIndex<4>\): Evaluating an Enthalpy of Formation Ozone, O₃(g), forms from oxygen, O₂(g), by an endothermic process. Ultraviolet radiation is the source of the energy that drives this reaction in the upper atmosphere. Assuming that both the reactants and products of the reaction are in their standard states, determine the standard enthalpy of formation, \(ΔH^\circ_\ce\) of ozone from the following information:

S olutio n \(ΔH^\circ_\ce\) is the enthalpy change for the formation of one mole of a substance in its standard state from the elements in their standard states. Thus, \(ΔH^\circ_\ce\) for O₃(g) is the enthalpy change for the reaction: \[\dfrac\ce(g)⟶\ce(g) \nonumber\] For the formation of 2 mol of O₃(g), \(ΔH^\circ_=+286\: \ce\). This ratio, \(\mathrm<\left(\dfrac<286\:kJ>\right)>\), can be used as a conversion factor to find the heat produced when 1 mole of O₃(g) is formed, which is the enthalpy of formation for O₃(g):

1. \(\ce_\)
2. \(\ce_\)

Solution

Remembering that \(ΔH^\circ_\ce\) reaction equations are for forming 1 mole of the compound from its constituent elements under standard conditions, we have:

Note: The standard state of carbon is graphite, and phosphorus exists as \(P_4\).

Write the heat of formation reaction equations for: